Defined benefit (DB) pension plans commonly distribute benefits as a monthly payment to an individual from the time of retirement until the individual’s death. This form of payment is called a single life annuity. Participants in DB plans often can choose from multiple types of annuities at retirement, but each of these options result in monthly payments. In some circumstances, benefits are distributed as a single payment rather than in monthly payments—this is known as a lump-sum distribution. This form of payment is generally permitted from plans when the “value” of the annuity is less than $5,000 (an IRS limit) or at higher values if the plan provides for this option. How do you determine the “value” of an annuity in which future payments are promised for an individual’s lifetime? This determination requires the skills of an actuary and is called a lump-sum conversion. The lump sum value of an annuity may also be called the actuarial present value of the annuity. Understanding this conversion requires understanding two concepts and a little mathematics. Each concept relies on a separate assumption about the future.
Present value
The first concept to understand is the time-value of money. One idea from the time-value of money is that having money today is more valuable than having money in the future. This means that a smaller amount of money today can be equivalent to a larger amount of money at a future date. Let’s consider an example. Imagine you committed to pay someone $1,000 one year from now, but decided you want to pay that person now rather than a year later. You can pay a smaller amount today that is equivalent to the entire promised amount if you are able to agree upon an assumed interest rate to apply. For example, $980.39 would grow to $1,000 in one year if it earned 2% interest. Thus, it would be fair to pay $980.39 today instead of $1,000 one year from now, assuming the recipient could earn 2% interest on that money. This amount is also called a present value (i.e., the value today). If the promised payment is further away, there is more time to grow and the “fair” amount today will be even less. If the $1,000 were promised five years from now and we continue to assume 2% interest, the amount today would be $905.73; if the amount were promised in 10 years, the amount today would be $820.35. This mathematical method is called discounting and can be seen in more detail below.
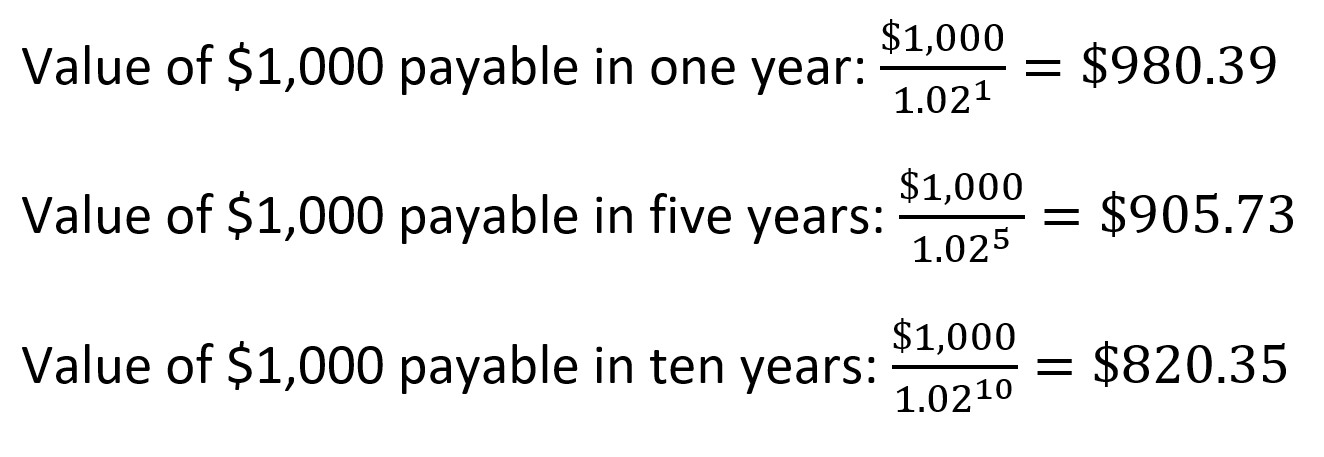
Actuaries apply this concept to a lump-sum conversion. Each future payment the individual could receive is reduced, or discounted, to the “fair” amount payable today, using interest rates provided by the IRS or specified by the plan.
Expected value
The second mathematical concept employed in a lump-sum conversion is probability, which addresses the question of how long a person is expected to live. Let’s begin with an example of using probability and then apply it to the lump-sum conversion. Imagine you agreed to a game in which someone would roll a single die and you would pay $1,000 if the person rolled a six and pay nothing for all other numbers. However, you decided you did not have time to play the game and want to just pay a “fair” amount instead. Because a die has six sides, we would expect the probability of winning the game to be one in six or 16.67%. Thus, the fair amount to pay, also known as the expected value, without playing the game would be $166.67. If you agreed to pay for rolling a one or a six, the probability would be two out of six or 33.33% and the fair payment would be $333.33.
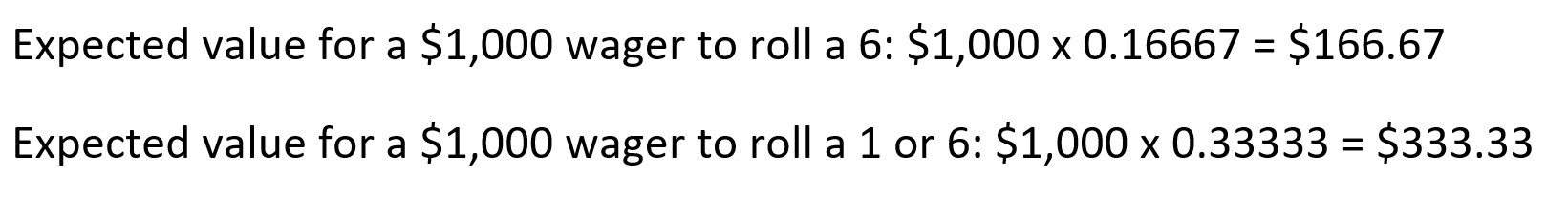
An expected value is calculated for each potential future payment when determining a lump-sum conversion. Similar to the fact that it is not possible to know if a person will actually roll a six on a die, it is not possible to know if a person will actually live and be eligible to receive a future payment. Actuaries rely on mortality tables to determine the probability that a person at a given age today is expected to be alive to receive a payment at any future date. Mortality tables contain the probability an individual will die at each age, and the probability of death is generally larger at older ages. Using the mortality assumption, the actuary is able to determine the “fair” or expected amount to pay a person today for any future payment.
Combining the concepts
These two concepts are combined to determine the “fair” value to pay today for each potential future annuity payment. As an example, suppose we wanted to determine the value of a single $1,000 annuity payment that is promised to be paid in 10 years, assuming a 5% interest rate and 92% probability that the individual will be alive to receive the payment. The “fair” amount to pay today is $564.80 after accounting for the timing of the payment (present value) and the probability the individual will be alive to receive the payment (expected value). The mathematics is shown below:
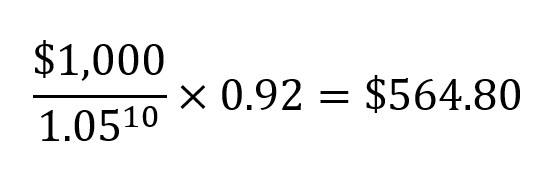
To determine the entire lump-sum value of the annuity, the calculation above is repeated for every potential future payment. Each result is summed together to determine the lump-sum distribution, or actuarial present value, of the annuity.
Disclosing assumptions
Actuaries must rely on these interest and mortality assumptions to convert an annuity to a lump-sum amount and the IRS mandates the use of certain assumptions in most cases. The assumptions used are typically disclosed with the lump-sum amount to inform the participant and to allow another actuary to replicate the conversion. The following is an example of a disclosure of the assumptions used to determine the lump-sum distribution under the minimum distribution requirements required by the IRS:
The assumptions used to determine the lump-sum distribution are the "2020 Applicable Mortality Table" as specified in IRS Notice 2019-26, and the segment interest rates of 2.04%, 3.09%, and 3.68%, which apply to the annuity payments due at specified periods in the future as required under Section 417(e) of the Internal Revenue Code for the month of November 2019.
Final thoughts
While the conversion of an annuity to a lump-sum distribution is complicated and requires an actuary, non-actuaries can understand and appreciate the principles and mathematical mechanics applied in the conversion. The calculation accounts for both the timing of the future payments (present value) and the probability the individual will be alive to receive the future payment (expected value) to arrive at a “fair” amount the individual should receive today in exchange for receiving annuity payments in the future.